Bevis for cosinussetningen: Forskjell mellom sideversjoner
m Teksterstatting – «<tex>» til «<math>» |
m Teksterstatting – «</tex>» til «</math>» |
||
| Linje 3: | Linje 3: | ||
[[Bilde:Bevcos111.PNG]] | [[Bilde:Bevcos111.PNG]] | ||
Bruker pytagoras på trekanten ADC:<p></p> | Bruker pytagoras på trekanten ADC:<p></p> | ||
<math>x^2 + h^2 = b^2 \Rightarrow h^2 = b^2 - x^2</ | <math>x^2 + h^2 = b^2 \Rightarrow h^2 = b^2 - x^2</math> | ||
<p></p> | <p></p> | ||
Bruker pytagoras på trekanten DBC:<p></p> | Bruker pytagoras på trekanten DBC:<p></p> | ||
<math>h^2 + (c-x)^2 = a^2</ | <math>h^2 + (c-x)^2 = a^2</math><p></p> | ||
Kombinerer de to utrykkene ved å sette inn for h i andre:<p></p> | Kombinerer de to utrykkene ved å sette inn for h i andre:<p></p> | ||
<math>b^2 - x^2 + c^2 - 2cx + x^2 =a^2 \\ | <math>b^2 - x^2 + c^2 - 2cx + x^2 =a^2 \\ | ||
a^2 = b^2 + c^2 -2cx</ | a^2 = b^2 + c^2 -2cx</math><p></p> | ||
Finner cosA: | Finner cosA: | ||
<p></p><math>cosA = \frac xb \Rightarrow x = b \cdot cosA</ | <p></p><math>cosA = \frac xb \Rightarrow x = b \cdot cosA</math><p></p>og får:<p></p> | ||
<math>a^2 = b^2 + c^2 -2bc cosA</ | <math>a^2 = b^2 + c^2 -2bc cosA</math> | ||
'''Stompvinklede:'''<p></p> | '''Stompvinklede:'''<p></p> | ||
| Linje 18: | Linje 18: | ||
Bruker pytagoras på trekanten DBC:<p></p> | Bruker pytagoras på trekanten DBC:<p></p> | ||
<math>a^2 = h^2 + (c+x)^2 \\ a^2 = h^2 + c^2 +2cx + x^2</ | <math>a^2 = h^2 + (c+x)^2 \\ a^2 = h^2 + c^2 +2cx + x^2</math><p></p> | ||
Bruker pytagoras på trekanten DAC:<p></p> | Bruker pytagoras på trekanten DAC:<p></p> | ||
<math>b^2 = x^2 + h^2 \Rightarrow h^2 = b^2 - x^2</ | <math>b^2 = x^2 + h^2 \Rightarrow h^2 = b^2 - x^2</math><p></p> Kombinere resultatene og får:<p></p> | ||
<math>a^2 = b^2 - x^2 + c^2 +2cx + x^2 \\ a^2 = b^2 + c^2 + 2cx</ | <math>a^2 = b^2 - x^2 + c^2 +2cx + x^2 \\ a^2 = b^2 + c^2 + 2cx</math><p></p> | ||
Fra enhetssirkelen har man at cosA = -cos(180-A). Da får man:<p></p> | Fra enhetssirkelen har man at cosA = -cos(180-A). Da får man:<p></p> | ||
<math>cos(180 - A) = - cosA = \frac xb \Rightarrow x = -bcosA </ | <math>cos(180 - A) = - cosA = \frac xb \Rightarrow x = -bcosA </math> som gir:<p></p> | ||
<math>a^2 = b^2 + c^2 - 2bccosA</ | <math>a^2 = b^2 + c^2 - 2bccosA</math> | ||
---- | ---- | ||
[[Category:bevis]][[Category:1T]][[Category:lex]] | [[Category:bevis]][[Category:1T]][[Category:lex]] | ||
Sideversjonen fra 5. feb. 2013 kl. 20:58
Man må vise at setningen gjelder både for spissvinklede og stompvinklede trekanter.
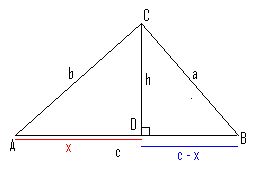
Spissvinklede:
Bruker pytagoras på trekanten ADC:
<math>x^2 + h^2 = b^2 \Rightarrow h^2 = b^2 - x^2</math>
Bruker pytagoras på trekanten DBC:
<math>h^2 + (c-x)^2 = a^2</math>
Kombinerer de to utrykkene ved å sette inn for h i andre:
<math>b^2 - x^2 + c^2 - 2cx + x^2 =a^2 \\
a^2 = b^2 + c^2 -2cx</math>
Finner cosA:
<math>cosA = \frac xb \Rightarrow x = b \cdot cosA</math>
og får:
<math>a^2 = b^2 + c^2 -2bc cosA</math>
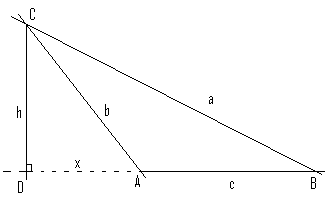
Stompvinklede:
Bruker pytagoras på trekanten DBC:
<math>a^2 = h^2 + (c+x)^2 \\ a^2 = h^2 + c^2 +2cx + x^2</math>
Bruker pytagoras på trekanten DAC:
<math>b^2 = x^2 + h^2 \Rightarrow h^2 = b^2 - x^2</math>
Kombinere resultatene og får:
<math>a^2 = b^2 - x^2 + c^2 +2cx + x^2 \\ a^2 = b^2 + c^2 + 2cx</math>
Fra enhetssirkelen har man at cosA = -cos(180-A). Da får man:
<math>cos(180 - A) = - cosA = \frac xb \Rightarrow x = -bcosA </math> som gir:
<math>a^2 = b^2 + c^2 - 2bccosA</math>