1T 2012 høst LØSNING: Forskjell mellom sideversjoner
Ingen redigeringsforklaring |
m Teksterstatting – «<tex>» til «<math>» |
||
| Linje 4: | Linje 4: | ||
== Oppgave 1 == | == Oppgave 1 == | ||
a = -2 og punkt. (3,0)<p></p> | a = -2 og punkt. (3,0)<p></p> | ||
< | <math>0 = -2 \cdot 3 + b \\ b= 6 \\ dvs: \\ y=-2x+6</tex> | ||
== Oppgave 2 == | == Oppgave 2 == | ||
< | <math>lg(2x+3) = 1 \\ 10^{lg(2x+3)} = 10^1 \\ 2x+3 =10 \\ x= \frac 72</tex> | ||
== Oppgave 3 == | == Oppgave 3 == | ||
< | <math>\frac{(2x)^3x^2}{2^5x^{-1}} = 2^{3-5}x^{3+2+1}= \frac{x^6}{4}</tex> | ||
== Oppgave 4 == | == Oppgave 4 == | ||
< | <math>\frac{x^2+6x+9}{x^2-9} = \frac{(x+3)(x+3)}{(x+3)(x-3)} = \frac{x+3}{x-3}</tex> | ||
== Oppgave 5 == | == Oppgave 5 == | ||
< | <math> (\sqrt2 + \sqrt8)^2 = 2+2\sqrt2\sqrt8+8 = 18</tex> | ||
== Oppgave 6 == | == Oppgave 6 == | ||
| Linje 18: | Linje 18: | ||
Nullpunkter: | Nullpunkter: | ||
f(x) = 0 <p></p> | f(x) = 0 <p></p> | ||
< | <math> x^2+2x-3 =0 \\ x= \frac{-2 \pm\sqrt{4+4 \cdot 3}}{2} \\ x=-3 \quad \vee \quad x=1</tex> | ||
=== b) === | === b) === | ||
< | <math>f'(x) = 2x+2 \\ f'(x) = 0 \\ x= -1 \\ f(-1)=-4</tex><p></p> f har et ekstremalpunkt i (-1,-4). Dette er et minimumspunkt da den deriverte er negativ for verdier mindre enn -1, og positiv for større verdier. | ||
=== c) === | === c) === | ||
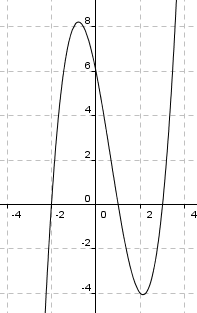
[[Fil:1T-host2012.png]] | [[Fil:1T-host2012.png]] | ||
== Oppgave 7 == | == Oppgave 7 == | ||
< | <math>(x+5)(x+3)-(x+5)(2x+7)=0 \\ (x+5)(x+3-2x-7)=0 \\ (x+5)=0 \quad \vee \quad -x-4=0 \\ x=-5 \quad \vee \quad x=-4</tex> | ||
== Oppgave 8 == | == Oppgave 8 == | ||
=== a) === | === a) === | ||
| Linje 31: | Linje 31: | ||
<td> </td> | <td> </td> | ||
<td>''' ''Bio'' '''</td> | <td>''' ''Bio'' '''</td> | ||
<td>''' ''< | <td>''' ''<math>\bar{Bio}</tex>'' '''</td> | ||
<td>'''Sum '''</td> | <td>'''Sum '''</td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td>''' ''Fys'' '''</td> | <td>''' ''Fys'' '''</td> | ||
<td> < | <td> <math>5</tex> </td> | ||
<td> < | <td> <math>7</tex> </td> | ||
<td> < | <td> <math>12</tex> </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td>''' ''< | <td>''' ''<math>\bar{Fys}</tex>'''</td> | ||
<td> < | <td> <math>9</tex> </td> | ||
<td> < | <td> <math>4</tex> </td> | ||
<td> < | <td> <math>13</tex> </td> | ||
</tr> | </tr> | ||
<tr> | <tr> | ||
<td> '''Sum''' </td> | <td> '''Sum''' </td> | ||
<td> < | <td> <math>14</tex> </td> | ||
<td> < | <td> <math>11</tex> </td> | ||
<td> < | <td> <math>25</tex> </td> | ||
</tr> | </tr> | ||
</table> | </table> | ||
=== b) === | === b) === | ||
< | <math>P(fys\quad og \quad bio) = \frac{5}{25} = \frac 15</tex> | ||
=== c) === | === c) === | ||
< | <math>P(fys\quad | \quad bio) = \frac{5}{14} </tex> | ||
== Oppgave 9 == | == Oppgave 9 == | ||
=== a) === | === a) === | ||
< | <math>SinA = \frac{12}{13} \\ CosA = \frac{5}{12}</tex> | ||
=== b) === | === b) === | ||
< | <math>(SinA)^2+(CosA)^2 = (\frac{12}{13})^2 + (\frac{5}{13})^2 = \frac{144+25}{169} = 1</tex> | ||
=== c) === | === c) === | ||
< | <math>a^2+c^2 = b^2 \\ \frac{a^2+c^2}{b^2}=1 \\ \frac{a^2}{b^2} + \frac{c^2}{b^2}= 1 \\ (\frac{a}{b})^2 + (\frac{c}{b})^2=1 \\ \frac ab = SinA \quad \wedge \quad \frac cb = CosA \\ (SinA)^2 + (CosA)^2 = 1 </tex> | ||
== Oppgave 10 == | == Oppgave 10 == | ||
< | <math> x^2 +x^2 = 16 \\ x= \sqrt 8</tex><p></p> Sidene i kvadratet har lengden kvadratroten av åtte. | ||
<p></p> | <p></p> | ||
Areal kvadrat = 8<p></p> | Areal kvadrat = 8<p></p> | ||
Areal sirkel =< | Areal sirkel =<math>\pi r^2 = \pi (\frac{\sqrt8}{2})^2 = 2\pi</tex> <p></p> | ||
Areal av skravert område blir: areal kvadrat - areal sirkel = < | Areal av skravert område blir: areal kvadrat - areal sirkel = <math>8-2\pi</tex> | ||
| Linje 81: | Linje 81: | ||
== Oppgave 1 == | == Oppgave 1 == | ||
=== a) === | === a) === | ||
< | <math> \frac{1}{R}= \frac{1}{R_1} + \frac{1}{R_2}\\ \frac{1}{R}=\frac{1}{5}+\frac{1}{7}\\ \frac{1}{R}=\frac{7}{35}+ \frac{5}{35}\\ \frac{1}{R} =\frac{12}{35}\\ 12R = 35 \\ R=\frac{35}{12} </tex> | ||
=== b) === | === b) === | ||
< | <math> | ||
R_2 = 2R_1 \\\frac{1}{R}=\frac{1}{R_1} +\frac{1}{2R_1}\\ \frac{1}{R}= \frac{2}{2R_1}+\frac{1}{R_1} \\ \frac{1}{R} = \frac{3}{2R_1} \\ 3R = 2R_1 \\ R = \frac{2}{3}R_1 </tex> | R_2 = 2R_1 \\\frac{1}{R}=\frac{1}{R_1} +\frac{1}{2R_1}\\ \frac{1}{R}= \frac{2}{2R_1}+\frac{1}{R_1} \\ \frac{1}{R} = \frac{3}{2R_1} \\ 3R = 2R_1 \\ R = \frac{2}{3}R_1 </tex> | ||
== Oppgave 2 == | == Oppgave 2 == | ||
| Linje 89: | Linje 89: | ||
[[Fil:1T-host2012-2.png]] | [[Fil:1T-host2012-2.png]] | ||
=== b) === | === b) === | ||
< | <math>f'(x) = 3x^2-4x-5 \\ f'(1)= 3-4-5 =-6 \\ f(1) = 1-2-5+6 =0 \\ 0 = -6 \cdot 1 + b \\ b= 6 \\ y= -6x+6</tex> | ||
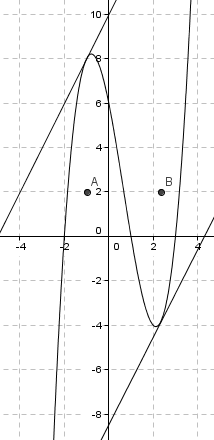
[[Fil:1T-host2012-2b.png]] | [[Fil:1T-host2012-2b.png]] | ||
=== c) === | === c) === | ||
| Linje 95: | Linje 95: | ||
[[Fil:1T-hoest2012-2c.png]]<p></p> | [[Fil:1T-hoest2012-2c.png]]<p></p> | ||
Finner at likningene til tangentene med stigningstall to er<p></p> | Finner at likningene til tangentene med stigningstall to er<p></p> | ||
< | <math>y = 2x +10 \\ y = 2x - 8,4</tex> | ||
== Oppgave 3 == | == Oppgave 3 == | ||
== a) == | == a) == | ||
< | <math>Cos \alpha = \frac {4}{11} \\ \alpha = 68,7^{\circ}</tex> | ||
== b) == | == b) == | ||
< | <math>h^2 = 11^2 - 4^2 \\ h = \sqrt{105} \approx 10,2</tex> | ||
| Linje 112: | Linje 112: | ||
Sannsynligheten for at de 10 første kundene betaler med kort:<p></p> | Sannsynligheten for at de 10 første kundene betaler med kort:<p></p> | ||
<p></p> | <p></p> | ||
< | <math>P = 0,6^{10} = 0,006 = 0,6%</tex> | ||
== b) == | == b) == | ||
| Linje 130: | Linje 130: | ||
== a) == | == a) == | ||
Velger 6 og 7.<p></p> | Velger 6 og 7.<p></p> | ||
< | <math>6+7+6^2 = 49 \\ 7^2 = 49</tex><p></p>Dette ser jo lovende ut.. | ||
== b) == | == b) == | ||
< | <math>n+(n+1)+ n^2 = (n+1)^2 \\ n^2 + 2n + 1 = (n+1)^2 \\ (n+1)^2 = (n+1)^2</tex> | ||
== Oppgave 6 == | == Oppgave 6 == | ||
== a) == | == a) == | ||
< | <math>(8-x)^2 =x^2+25 \\ 64-16x+x^2 = x^2+25 \\ -16x = -39 \\ x=2,4</tex> | ||
== b) == | == b) == | ||
< | <math> a^2 = b^2 + c^2 - 2bc cosA \\ x^2 = 25+64 - 16x + x^2 - 2\cdot 5 \cdot (8-x) cos 30^{\circ} \\ 7,33x = 19,7 \\ x = 2,7</tex> | ||
== c) == | == c) == | ||
< | <math>\frac{SinE}{5,3} = \frac{Sin 30^{\circ}}{2,7} \\ E = 79^{\circ} </tex> | ||
== Oppgave 7 == | == Oppgave 7 == | ||
== a) == | == a) == | ||
< | <math> 4x+h = 30 \\ h = 30-4x \\ Dersom \quad h = 0 \Rightarrow x = \frac{30}{4} = 7,5 \\ 0<x<7,5</tex> | ||
== b) == | == b) == | ||
< | <math>O(x) = x^2 + 4x(30-4x) \\ O(x)=x^2 + 120x - 16x^2 \\ O(x)= -15x^2+120x</tex> | ||
== c) == | == c) == | ||
< | <math>O'(x)= -30x+120 \\ O'(x) =0 \\ \Downarrow \\ -30x+120 =0 \\ x =4 </tex><p></p>Fire desimeter gir den største overflanten. Da er overflaten:<p></p> | ||
< | <math>O(4) = -15 \cdot 4^2 + 120 \cdot 4 = 240</tex><p></p> | ||
Da er overflaten 240 kvadratdesimeter. | Da er overflaten 240 kvadratdesimeter. | ||
Sideversjonen fra 5. feb. 2013 kl. 20:50
DEL EN
Oppgave 1
a = -2 og punkt. (3,0)
<math>0 = -2 \cdot 3 + b \\ b= 6 \\ dvs: \\ y=-2x+6</tex>
Oppgave 2
<math>lg(2x+3) = 1 \\ 10^{lg(2x+3)} = 10^1 \\ 2x+3 =10 \\ x= \frac 72</tex>
Oppgave 3
<math>\frac{(2x)^3x^2}{2^5x^{-1}} = 2^{3-5}x^{3+2+1}= \frac{x^6}{4}</tex>
Oppgave 4
<math>\frac{x^2+6x+9}{x^2-9} = \frac{(x+3)(x+3)}{(x+3)(x-3)} = \frac{x+3}{x-3}</tex>
Oppgave 5
<math> (\sqrt2 + \sqrt8)^2 = 2+2\sqrt2\sqrt8+8 = 18</tex>
Oppgave 6
a)
Nullpunkter:
f(x) = 0
<math> x^2+2x-3 =0 \\ x= \frac{-2 \pm\sqrt{4+4 \cdot 3}}{2} \\ x=-3 \quad \vee \quad x=1</tex>
b)
<math>f'(x) = 2x+2 \\ f'(x) = 0 \\ x= -1 \\ f(-1)=-4</tex>
f har et ekstremalpunkt i (-1,-4). Dette er et minimumspunkt da den deriverte er negativ for verdier mindre enn -1, og positiv for større verdier.
c)
Oppgave 7
<math>(x+5)(x+3)-(x+5)(2x+7)=0 \\ (x+5)(x+3-2x-7)=0 \\ (x+5)=0 \quad \vee \quad -x-4=0 \\ x=-5 \quad \vee \quad x=-4</tex>
Oppgave 8
a)
| Bio | <math>\bar{Bio}</tex> | Sum | |
| Fys | <math>5</tex> | <math>7</tex> | <math>12</tex> |
| <math>\bar{Fys}</tex> | <math>9</tex> | <math>4</tex> | <math>13</tex> |
| Sum | <math>14</tex> | <math>11</tex> | <math>25</tex> |
b)
<math>P(fys\quad og \quad bio) = \frac{5}{25} = \frac 15</tex>
c)
<math>P(fys\quad | \quad bio) = \frac{5}{14} </tex>
Oppgave 9
a)
<math>SinA = \frac{12}{13} \\ CosA = \frac{5}{12}</tex>
b)
<math>(SinA)^2+(CosA)^2 = (\frac{12}{13})^2 + (\frac{5}{13})^2 = \frac{144+25}{169} = 1</tex>
c)
<math>a^2+c^2 = b^2 \\ \frac{a^2+c^2}{b^2}=1 \\ \frac{a^2}{b^2} + \frac{c^2}{b^2}= 1 \\ (\frac{a}{b})^2 + (\frac{c}{b})^2=1 \\ \frac ab = SinA \quad \wedge \quad \frac cb = CosA \\ (SinA)^2 + (CosA)^2 = 1 </tex>
Oppgave 10
<math> x^2 +x^2 = 16 \\ x= \sqrt 8</tex>
Sidene i kvadratet har lengden kvadratroten av åtte.
Areal kvadrat = 8
Areal sirkel =<math>\pi r^2 = \pi (\frac{\sqrt8}{2})^2 = 2\pi</tex>
Areal av skravert område blir: areal kvadrat - areal sirkel = <math>8-2\pi</tex>
DEL TO
Oppgave 1
a)
<math> \frac{1}{R}= \frac{1}{R_1} + \frac{1}{R_2}\\ \frac{1}{R}=\frac{1}{5}+\frac{1}{7}\\ \frac{1}{R}=\frac{7}{35}+ \frac{5}{35}\\ \frac{1}{R} =\frac{12}{35}\\ 12R = 35 \\ R=\frac{35}{12} </tex>
b)
<math> R_2 = 2R_1 \\\frac{1}{R}=\frac{1}{R_1} +\frac{1}{2R_1}\\ \frac{1}{R}= \frac{2}{2R_1}+\frac{1}{R_1} \\ \frac{1}{R} = \frac{3}{2R_1} \\ 3R = 2R_1 \\ R = \frac{2}{3}R_1 </tex>
Oppgave 2
a)
b)
<math>f'(x) = 3x^2-4x-5 \\ f'(1)= 3-4-5 =-6 \\ f(1) = 1-2-5+6 =0 \\ 0 = -6 \cdot 1 + b \\ b= 6 \\ y= -6x+6</tex>
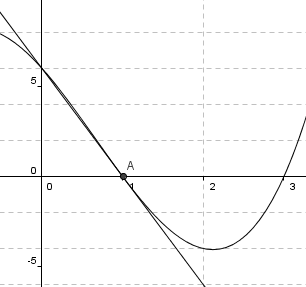
c)
Bruker Geogebra:
Finner at likningene til tangentene med stigningstall to er
<math>y = 2x +10 \\ y = 2x - 8,4</tex>
Oppgave 3
a)
<math>Cos \alpha = \frac {4}{11} \\ \alpha = 68,7^{\circ}</tex>
b)
<math>h^2 = 11^2 - 4^2 \\ h = \sqrt{105} \approx 10,2</tex>
Oppgave 4
a)
Sannsynlighet for å betale med kort P(kort) = 0,6
Sannsynligheten for at de 10 første kundene betaler med kort:
<math>P = 0,6^{10} = 0,006 = 0,6%</tex>
b)
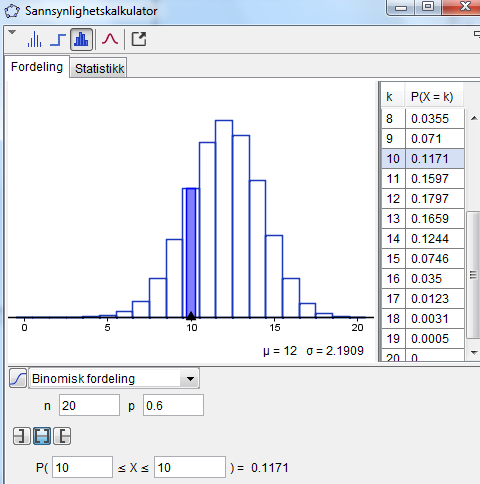
Sannsynligheten for at 10 av de første 20 bilene betaler med kort.
Sannsynligheten er 11,7%
c)
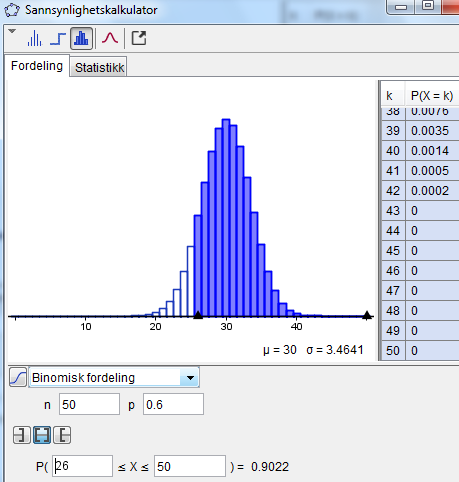
Sannsynligheten for at mer enn 25 av de 50 første bilene betaler med kort:
Sannsynligheten er 90,2%
Oppgave 5
a)
Velger 6 og 7.
<math>6+7+6^2 = 49 \\ 7^2 = 49</tex>
Dette ser jo lovende ut..
b)
<math>n+(n+1)+ n^2 = (n+1)^2 \\ n^2 + 2n + 1 = (n+1)^2 \\ (n+1)^2 = (n+1)^2</tex>
Oppgave 6
a)
<math>(8-x)^2 =x^2+25 \\ 64-16x+x^2 = x^2+25 \\ -16x = -39 \\ x=2,4</tex>
b)
<math> a^2 = b^2 + c^2 - 2bc cosA \\ x^2 = 25+64 - 16x + x^2 - 2\cdot 5 \cdot (8-x) cos 30^{\circ} \\ 7,33x = 19,7 \\ x = 2,7</tex>
c)
<math>\frac{SinE}{5,3} = \frac{Sin 30^{\circ}}{2,7} \\ E = 79^{\circ} </tex>
Oppgave 7
a)
<math> 4x+h = 30 \\ h = 30-4x \\ Dersom \quad h = 0 \Rightarrow x = \frac{30}{4} = 7,5 \\ 0<x<7,5</tex>
b)
<math>O(x) = x^2 + 4x(30-4x) \\ O(x)=x^2 + 120x - 16x^2 \\ O(x)= -15x^2+120x</tex>
c)
<math>O'(x)= -30x+120 \\ O'(x) =0 \\ \Downarrow \\ -30x+120 =0 \\ x =4 </tex>
Fire desimeter gir den største overflanten. Da er overflaten:
<math>O(4) = -15 \cdot 4^2 + 120 \cdot 4 = 240</tex>
Da er overflaten 240 kvadratdesimeter.