Trigonometriske identiteter: Forskjell mellom sideversjoner
Ingen redigeringsforklaring |
Ingen redigeringsforklaring |
||
| Linje 17: | Linje 17: | ||
<center> | |||
{| class="wikitable" style="background-color:#FFFFFF;text-align:center" | |||
|+ Hver av de trigonometriske funksjonene uttrykt ved de andre fem..<ref>Abramowitz and Stegun, p. 73, 4.3.45</ref> | |||
! Uttrykt ved | |||
! scope="col" | <math> \sin v\!</math> | |||
! scope="col" | <math> \cos v\!</math> | |||
! scope="col" | <math> \tan v!</math> | |||
! scope="col" | <math> \csc v\!</math> | |||
! scope="col" | <math> \sec v\!</math> | |||
! scope="col" | <math> \cot v\!</math> | |||
|- | |||
! <math> \sin v =\!</math> | |||
| <math> \sin v\ </math> | |||
| <math>\pm\sqrt{1 - \cos^2 v}\! </math> | |||
| <math>\pm\frac{\tan v}{\sqrt{1 + \tan^2 v}}\! </math> | |||
| <math> \frac{1}{\csc v}\! </math> | |||
| <math>\pm\frac{\sqrt{\sec^2 v - 1}}{\sec v}\! </math> | |||
| <math>\pm\frac{1}{\sqrt{1 + \cot^2 v}}\! </math> | |||
|- | |||
! <math> \cos \theta =\!</math> | |||
| <math>\pm\sqrt{1 - \sin^2\theta}\! </math> | |||
| <math> \cos \theta\! </math> | |||
| <math>\pm\frac{1}{\sqrt{1 + \tan^2 \theta}}\! </math> | |||
| <math>\pm\frac{\sqrt{\csc^2 \theta - 1}}{\csc \theta}\! </math> | |||
| <math> \frac{1}{\sec \theta}\! </math> | |||
| <math>\pm\frac{\cot \theta}{\sqrt{1 + \cot^2 \theta}}\! </math> | |||
|- | |||
! <math> \tan \theta =\!</math> | |||
| <math>\pm\frac{\sin \theta}{\sqrt{1 - \sin^2 \theta}}\! </math> | |||
| <math>\pm\frac{\sqrt{1 - \cos^2 \theta}}{\cos \theta}\! </math> | |||
| <math> \tan \theta\! </math> | |||
| <math>\pm\frac{1}{\sqrt{\csc^2 \theta - 1}}\! </math> | |||
| <math>\pm\sqrt{\sec^2 \theta - 1}\! </math> | |||
| <math> \frac{1}{\cot \theta}\! </math> | |||
|- | |||
! <math> \csc \theta =\!</math> | |||
| <math> \frac{1}{\sin \theta}\! </math> | |||
| <math>\pm\frac{1}{\sqrt{1 - \cos^2 \theta}}\! </math> | |||
| <math>\pm\frac{\sqrt{1 + \tan^2 \theta}}{\tan \theta}\! </math> | |||
| <math> \csc \theta\! </math> | |||
| <math>\pm\frac{\sec \theta}{\sqrt{\sec^2 \theta - 1}}\! </math> | |||
| <math>\pm\sqrt{1 + \cot^2 \theta}\! </math> | |||
|- | |||
! <math> \sec \theta =\!</math> | |||
| <math>\pm\frac{1}{\sqrt{1 - \sin^2 \theta}}\! </math><center> | |||
| <math> \frac{1}{\cos \theta}\! </math> | |||
| <math>\pm\sqrt{1 + \tan^2 \theta}\! </math> | |||
| <math>\pm\frac{\csc \theta}{\sqrt{\csc^2 \theta - 1}}\! </math> | |||
| <math> \sec \theta\! </math> | |||
| <math>\pm\frac{\sqrt{1 + \cot^2 \theta}}{\cot \theta}\! </math> | |||
|- | |||
! <math> \cot \theta =\!</math> | |||
| <math>\pm\frac{\sqrt{1 - \sin^2 \theta}}{\sin \theta}\! </math> | |||
| <math>\pm\frac{\cos \theta}{\sqrt{1 - \cos^2 \theta}}\! </math> | |||
| <math> \frac{1}{\tan \theta}\! </math> | |||
| <math>\pm\sqrt{\csc^2 \theta - 1}\! </math> | |||
| <math>\pm\frac{1}{\sqrt{\sec^2 \theta - 1}}\! </math> | |||
| <math> \cot \theta\! </math> | |||
|}</center> | |||
Sideversjonen fra 15. jul. 2015 kl. 12:24
Nedenfor følger en rekke trigonometriske identiteter. Noen er pensum i norsk skole (R2), andre ikke. Vi mener det er riktig å vise alle, da noen av dere kan komme til å studere i land der disse er pensum. Ha oss tilgitt, ikke alt er like lett å vise grafisk, men vi prøver så godt vi kan.
Definisjoner:
Det finnes mange trigonometriske identiteter. Her er noen av dem.
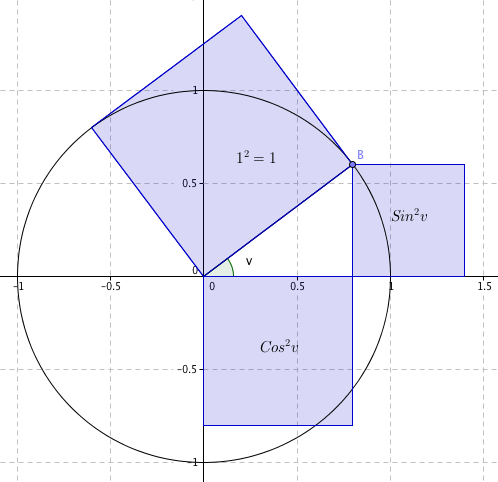
$sin^2v + cos^2v = 1$
Relasjonen fremkommer ved å anvende Pytagoras direkte i enhetssirkelen.
$ \quad \quad tan^2v + 1 = sec^2v\quad \quad cot^2v+1 = csc^2v$
| Uttrykt ved | <math> \sin v\!</math> | <math> \cos v\!</math> | <math> \tan v!</math> | <math> \csc v\!</math> | <math> \sec v\!</math> | <math> \cot v\!</math> |
|---|---|---|---|---|---|---|
| <math> \sin v =\!</math> | <math> \sin v\ </math> | <math>\pm\sqrt{1 - \cos^2 v}\! </math> | <math>\pm\frac{\tan v}{\sqrt{1 + \tan^2 v}}\! </math> | <math> \frac{1}{\csc v}\! </math> | <math>\pm\frac{\sqrt{\sec^2 v - 1}}{\sec v}\! </math> | <math>\pm\frac{1}{\sqrt{1 + \cot^2 v}}\! </math> |
| <math> \cos \theta =\!</math> | <math>\pm\sqrt{1 - \sin^2\theta}\! </math> | <math> \cos \theta\! </math> | <math>\pm\frac{1}{\sqrt{1 + \tan^2 \theta}}\! </math> | <math>\pm\frac{\sqrt{\csc^2 \theta - 1}}{\csc \theta}\! </math> | <math> \frac{1}{\sec \theta}\! </math> | <math>\pm\frac{\cot \theta}{\sqrt{1 + \cot^2 \theta}}\! </math> |
| <math> \tan \theta =\!</math> | <math>\pm\frac{\sin \theta}{\sqrt{1 - \sin^2 \theta}}\! </math> | <math>\pm\frac{\sqrt{1 - \cos^2 \theta}}{\cos \theta}\! </math> | <math> \tan \theta\! </math> | <math>\pm\frac{1}{\sqrt{\csc^2 \theta - 1}}\! </math> | <math>\pm\sqrt{\sec^2 \theta - 1}\! </math> | <math> \frac{1}{\cot \theta}\! </math> |
| <math> \csc \theta =\!</math> | <math> \frac{1}{\sin \theta}\! </math> | <math>\pm\frac{1}{\sqrt{1 - \cos^2 \theta}}\! </math> | <math>\pm\frac{\sqrt{1 + \tan^2 \theta}}{\tan \theta}\! </math> | <math> \csc \theta\! </math> | <math>\pm\frac{\sec \theta}{\sqrt{\sec^2 \theta - 1}}\! </math> | <math>\pm\sqrt{1 + \cot^2 \theta}\! </math> |
| <math> \sec \theta =\!</math> | <math>\pm\frac{1}{\sqrt{1 - \sin^2 \theta}}\! </math> |
<math> \frac{1}{\cos \theta}\! </math> | <math>\pm\sqrt{1 + \tan^2 \theta}\! </math> | <math>\pm\frac{\csc \theta}{\sqrt{\csc^2 \theta - 1}}\! </math> | <math> \sec \theta\! </math> | <math>\pm\frac{\sqrt{1 + \cot^2 \theta}}{\cot \theta}\! </math> |
| <math> \cot \theta =\!</math> | <math>\pm\frac{\sqrt{1 - \sin^2 \theta}}{\sin \theta}\! </math> | <math>\pm\frac{\cos \theta}{\sqrt{1 - \cos^2 \theta}}\! </math> | <math> \frac{1}{\tan \theta}\! </math> | <math>\pm\sqrt{\csc^2 \theta - 1}\! </math> | <math>\pm\frac{1}{\sqrt{\sec^2 \theta - 1}}\! </math> | <math> \cot \theta\! </math> |
$cos(u-v) = cos(u)\cdot cos(v)+sin(u) \cdot sin(v) \quad \quad cos(u + v) = cos(u)\cdot cos(v)-sin(u)\cdot sin(v) \\ sin(u - v) = sin(u)\cdot cos(v)-cos(u)\cdot sin(v) \quad \quad sin(u + v) = sin(u)\cdot cos(v)+cos(u)\cdot sin(v)$
<math>sin(2u) = 2sin(u) \cdot cos(u) </math>
<math>cos(2u) = cos^2 (u) - sin^2 (u) </math>
<math>1 + cos(2u) = 2 cos^2 (u)</math>
<math>1 - cos(2u) = 2 sin^2 (u)</math>
Dersom u + v = 180° har vi at Sin v = sin u og cos v = -cos u